Is it possible to fully understand Gödel's incompleteness theorem in 15 minutes?
Of course not! But amazingly enough, 15 minutes are enough to understand one of the key ideas behind its proof. Prof. Marcus du Sautoy, mathematician at Oxford University, managed to explain in this short Numberphile video what Gödel numbers are and how they show the way towards proving that any system of axioms is incomplete.
The first time I heard about Gödel's incompleteness theorem was during my undergraduate studies in mathematics, during an introductory course in mathematical logic. I still remember that when we asked the lecturer to tell us about this theorem the response was that it is too complicated to discuss in detail. But was it really so complicated that not even the basic ideas behind it could be discussed during such an introductory course?
Many times mathematicians are reluctant to explain their own work because of the technicalities that accompany their work. Trying to figure out which technicalities are essential is definitely not an easy task, but also not an impossible one. On the other hand, when trying to write an article aiming at popularizing some aspect or result from mathematics we tend to use allegories in order to make the explanation more accessible. The general tendency is to try and reduce the actual math, which for me is similar to trying to learn a sport without actually practicing it. The same happens with math, you can't learn math by only watching a video, you have to try it out with pen and paper. But sometimes a video can give you the right intuition and motivation to start exploring the math.
This Numberphile video, and also a much more detailed and elaborate video made by Veritasium, prove that it is indeed possible to explain some ideas from Gödel's work without needing all the formal details. The amazing is that they don't rely on some allegory or vague simplification, but they show the actual mathematical method Gödel developed! Showing the actual math makes it much more fun to watch.
Of course to get a deep understanding of Gödel's work much more effort is needed. Watching these videos gave me some confidence and motivation to read further on Gödel's work and work out some examples of Gödel-numbers on my own. A good starting point is maybe the book "On Formally Undecidable Propositions of Principia Mathematica and Related Systems" by Dover publications.
I want to conclude this short post with something I read in a translation of Gödel's article, written by Prof. Richard Bevan Braithwaite:
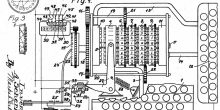
"Gödel’s novel metamathematical method is that of attaching numbers to the signs, to the series of signs (formulae) and to the series of series of signs (‘proof-schemata’) which occur in his formal system. Just as Descartes invented co-ordinate geometry by assigning number-pairs to the points of plane Euclidean geometry, so Gödel invented what might be called co-ordinate metamathematics by assigning numbers to the basic signs, series of basic signs, series of series of basic signs (all of which I shall for convenience lump together under the generic term string) which form an essential part of the subject-matter of metamathematics. Descartes proved geometrical theorems about points by proving algebraic theorems about numbers; Gödel established metamathematical results about the strings of his formal system by considering numbers coordinated with the strings."
During the last decades, many resources have been invested in quantum computing. This research field has grown immensely and amazing results have been obtained. The plans for the future are highly ambitious, but at the same time, there are serious questions that need to be addressed.
A mathematician once told me that problems that are very simple to state can be very deceiving, and sometimes turn out to be extremely difficult to solve. One such problem was the Four Color Problem.
One of the main building blocks of modern AI-tools are artificial neural networks, abstract models inspired by the structure and functions of biological neural networks which enable machines to "learn". In this article, I will discuss some thoughts on this topic.
I was very excited and interested when I heard some months ago about ChatGPT and what it can do. Both as a mathematician and as a teacher I was very curious about the responses it would give to my questions.
On a quiet afternoon, professor Meth is working in her office in Leiden on some tantalizing mathematics problems. Suddenly, someone knocking on her door nervously disrupts the silence.
